Problem Statement
The question asks us to identify the following absolute value equation from the options:

Solution
To identify the correct absolute value equation, we need to know two things:
1. The vertex of the absolute value equation:
The vertex is illustrated below:
The vertex of an absolute value equation can be gotten from the equation itself as shown below:
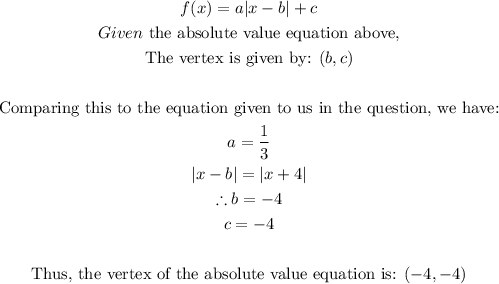
2. The orientation of the graph:
This means whether or not the graph tails are pointing in a certain direction. In the figure above, the tails of graph A are pointing upwards, while in graph B, the tails are pointing downwards.
To know what direction an absolute value equation points, we have:
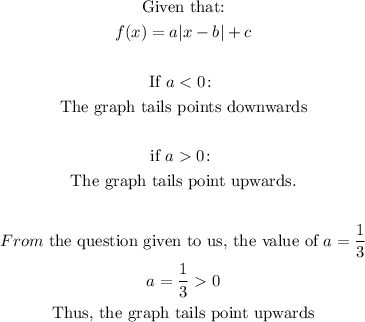
Given the two deductions drawn from above, we expect:
1. The graph to have its vertex in the bottom left (or 3rd quadrant) of the graph.
2. The tails of the graph are pointing upwards.
We can plot the graph using a graphing calculator as shown below: