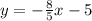Question

Write the equation of a line perpendicular to 5x - 8y = - 3 that passes through the point (-5,3).

Answer 1

To find the perpendicular line, first, let's rewrite this line in slope-intercept form. The slope-intercept form is

`y=mx+b`

Where m represents the slope and b the y-intercept.

Rewritting our line equation on this form, we have

`\begin{gathered} 5x-8y=-3 \\ -8y=-5x-3 \\ 8y=5x+3 \\ y=(5)/(8)x+(3)/(8) \end{gathered}`

The slope of the perpendicular line is minus the inverse of the slope of our line.

`m_(\perp)=-((5)/(8))^(-1)=-(8)/(5)`

Then, this means the perpendicular line have the form

`y=-(8)/(5)x+b`

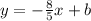
To find the coefficient b, we can evaluate the point we know that belongs to this line.

`\begin{gathered} (3)=-(8)/(5)(-5)+b \\ 3=8+b \\ b=3-8 \\ b=-5 \end{gathered}`

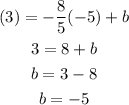
Our perpendicular line is

`y=-(8)/(5)x-5`