ANSWER
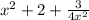
Step-by-step explanation
We want to rewrite the given expression:
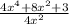
in the form of a quotient, a remainder, and a divisor.
Since there is only one term in the divisor, we can rewrite the expression by dividing each term in the numerator by the divisor as follows:
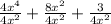
Now, simplify each term:
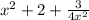
That is the answer in the form of a quotient, a remainder, and a divisor.