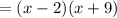EXPLANATION
Given the equation:
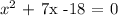
We can apply the quadratic equations formula as shown as follows:
Break the expression into groups:
For:

Find u,v such that u*v = a*c and u+v = b and group into (ax^2+ux)+(vx+c)
a=1, b=7, c=-18
u*v=-18, u+v = 7
Find the primer factors of 18:
18 / 2 = 9
9 / 3 = 3
2,3 are all prime numbers, therefore no further factorization is possible.
Multiply the prime factors of 18: 6,9
Add the prime factors: 2,3
Add 1 and the number 18 itself
1, 18
The factors of 18:
1, 2, 3 , 6, 9, 18
Negative factors of 18:
Multiply the factors by -1 to get the negative factors:
-1, -2, -3, -6, -9, -18
For every two factors such that u*v=-18 , check if u+v = 7:

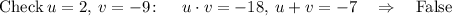
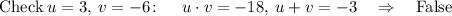
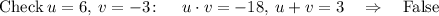

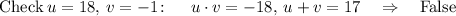
u=9, v=-2
Group into:
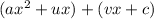
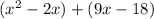
Factor out x from x^2 -2x
x^2 -2x
Factor out common term x:
=x(x-2)
Factor out 9 from 9x - 18:
Rewrite 18 as 2*9:
9x - 9*2
Factor out common term 9:
9(x-2)
=x(x-2) + 9(x-2)
Factor out common term x-2:
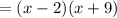
The solution to the quadratic equation x^2 + 7x - 18 = 0 applying the factorizing method is: