Given:
Vector A = 63.5 m at 90 degrees
Vector B = 101 m at 57.0 degrees
Let's find the direction of the sum of these two vectors.
To find the direction, let's first find the x- and y-components of the vectors.
• Vector A:
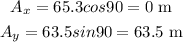
• Vector B:
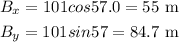
For the sum of components, we have:
x = Ax + Bx = 0 + 55 = 55 m
y = Ay + By = 63.5 + 84.7 = 148.2 m
Now, to find the direction of the sum, we have:
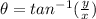
Plug in the values and solve for θ.
We have:
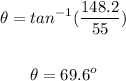
Therefore, the direction of the sum of the vectors is 69.6 degrees.
ANSWER:
69.6 degrees.