Question

o Watch help videoIf ST = 107, TU = 102, and W X = 51, find the length of VW. Roundyour answer to the nearest tenth if necessary. Figures are not necessarilydrawn to scale.wv59Submit AnswerAnswer: VW =attempt 1 out of 2

Answer 1

We have two triangle UTS, and WXV, they are simillar because all of their angles are equal. This is demonstrated by the fact the angle U and W are:

`\begin{gathered} \measuredangle U=180-67-54=59 \\ \measuredangle W=180-54-59=67 \end{gathered}`

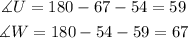
This means that every corresponding sides of the triangles are related by the same constant. We can use this fact to calculate VW, as shown below:

`\begin{gathered} (VW)/(WX)=(ST)/(TU) \\ (VW)/(51)=(107)/(102) \\ VW=(51\cdot107)/(102) \\ VW=53.5 \end{gathered}`

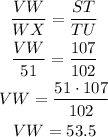
The side VW has a length of 53.5