Question

A box of mass 5.7 kg slides across a floor and comes to a complete stop. If it’s initial speed was 10 km/h and μk = 0.34, find:A. The friction acting on the boxB. The acceleration of the boxC. The distance travelled by the box before stoppingD. The time it took to stop

Answer 1

Given:

mass, m = 5.7 kg

Initial velocity = 10 km/h

Coefficient of kinetic friction, μk = 0.34

Final velocity = 0 km/h

Let's solve for the following:

• (A). The friction acting on the box.

To find the force acting on the box, apply the formula:

`f=mg*\mu k`

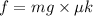
Where:

• m = 5.7 kg

,

• g is acceleration due to gravity = 9.8 m/s²

,

• μk = 0.34

Thus, we have:

`\begin{gathered} f=5.7*9.8*0.34 \\ \\ f=19\text{ N} \end{gathered}`

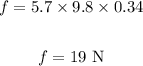
The friction acting on the best is 19 N.

• (B). The acceleration of the box.

Here, the force is provided by the frictional force. Hence, the friction acting on the box is equal to the frictional force.

To find the acceleration, apply the formula:

`F=ma`

Where:

F = 19 N

m = 0.34

Let's solve for a:

`\begin{gathered} a=(F)/(m) \\ \\ a=(19)/(5.7) \\ \\ a=3.3m/s^2 \end{gathered}`

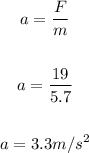
The acceleration of the box is -3.3 m/s².

It is negative because it is deccelerating.

• (c). The distance travelled by the box before stopping

To find the distance traveled by the box, apply the third equation of motion:

`v^2_f-v^2_i=2ad`

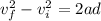
Where d is the distance.

vf = 0 m/s

vi = 10 km/h

a = -3.3 m/s^2

Rewrite the formula for d:

`d=(v^2_f-v^2_i)/(2a)`

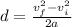
Convert the initial velocity from km/h to m/s:

Where:

1 m/s = 3.6 km/h

`10\text{ km/h=}(10)/(3.6)=2.78\text{ m/s}`

Thus, we have:

`\begin{gathered} d=(0^2-2.78^2)/(2(-3.3)) \\ \\ d=1.18\text{ m} \end{gathered}`

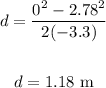
The distance is 1.18 meters.

(d). The time it took to stop.

To find the time, apply the formula:

`\begin{gathered} v_f=v_i+at \\ \\ t=(v_f-v_i)/(a) \end{gathered}`

Thus, we have:

`\begin{gathered} t=(0-2.78)/(-3.3) \\ \\ t=0.84\text{ s} \end{gathered}`

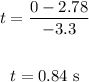
The time is 0.84 seconds.

ANSWER:

• A. 19 N

,

• B. -3.3 m/s²

,

• C. 1.18 m

,

• D. 0.84 s