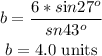Question

I need help on this practice problem for a completion grade it’s the last question for this practice assignment

Answer 1

we know that

A=110 degrees

B =27 degrees

c=6

step 1

Find out the measure of angle C

Remember that

The sum of the interior angles in any triangle must be equal to 180 degrees

so

A+B+C=180 degrees

substitute given values

110+27+C=180

137+C=180

C=180-137

C=43 degrees

step 2

Find out the length side a

Applying the law of sines

`(c)/(sinC)=(a)/(sinA)`

substitute given values

`(6)/(s\imaginaryI n43^o)=(a)/(s\imaginaryI n110^o)`

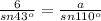
solve for a

`\begin{gathered} a=\frac{6*s\mathrm{i}n110^o}{s\imaginaryI n43^o} \\ a=8.3\text{ units} \end{gathered}`

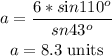
step 3

Find out the length of side b

Apply the law of sines

`(c)/(s\imaginaryI nC)=(a)/(s\imaginaryI nA)`

substitute given values

`(6)/(s\imaginaryI n43^o)=(b)/(s\imaginaryI n27^o)`

solve for b

`\begin{gathered} b=\frac{6*s\mathrm{i}n27^o}{s\imaginaryI n43^o} \\ b=4.0\text{ units} \end{gathered}`