Question

Hi, can you help me to solve exercise #2 please

Answer 1

N 2

we have

f(x)=-x^2-4x+12

this is a vertical parabola open downward (because the leading coefficient is negative)

The vertex represents the maximum

Convert the given equation into vertex form

f(x)=a(x-h)^2+k

where

(h,k) is the vertex

The axis of symmetry is equal to x=h

so

f(x)=-x^2-4x+12

factor -1

f(x)=-(x^2+4x)+12

Complete the square

f(x)=-(x^2+4x+2^2-2^2)+12

f(x)=-(x+2)^2+16

the vertex is (-2,16)

The axis of symmetry is x=-2

Find out the y-intercept (value of f(x) when the value of x=0)

For x=0

f(x)=-(0)^2-4(0)+12

f(x)=12

The y-intercept is (0,12)

Graph the function

we have the points

(-2,16) vertex

(0,12) y-intercept

Find out te x-intercepts (values of x when the value of f(x)=0

-x^2-4x+12=0

solve the quadratic equation using the formula

a=-1

b=-4

c=12

substitute

`x=\frac{-(-4)\pm\sqrt[]{-4^2-4(-1)(12)}}{2(-1)}`
`x=(4\pm8)/(-2)`

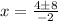
The values of x are

x=