Given: The function below:
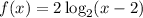
The function f(x) is logarithmic function with a vertical asymptote
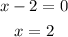
Calculate the domain
From the graph shown the domain is
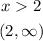
From the graph, the range is
[tex]\begin{gathered} -\infty
HenceThe function f(x) is logarithmic function with a vertical asymptote of 2. The range of the function is (- ∞, ∞) and it is increasing on its domain of (2, ∞)