Question

Write an expression for the perimeter of the figure shown.

Answer 1

The perimeter of a figure is the boundary of its surface, in this case, it is:

`P=(x+1)+x+x+(x+3)+x+(x+1)+(x+3)`

I will edit the image to help you understand

This is quite improvised but it'll help you to understand how the sides are related.

So, continuing with the calculation:

`\begin{gathered} P=(x+1)+x+x+(x+3)+x+(x+1)+(x+3) \\ \Rightarrow P=x+1+2x+x+3+x+x+1+x+3 \\ \Rightarrow P=7x+8 \end{gathered}`

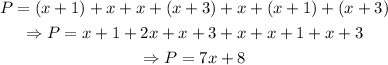
So, the answer is: Perimeter = 7x+8