The above diagram shows this situation. We have to find the values of x and y
Definition of sine

Applying this definition to the angle of 50°, and solving for x:
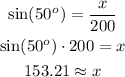
Definition of cosine
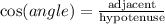
Applying this definition to the angle of 50°, and solving for y:
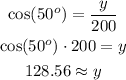
Answer: The car traveled 153.21 miles east and 128.56 miles north