According to the question,
Initial velocity of the car (
 ) = 0 m/s
) = 0 m/s
Final velocity of the car (
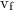 ) = 28 m/s
) = 28 m/s
Time of uniform acceleration (t) = 5.6 s
a. By using
 kinematic equation and substituting value we get:
kinematic equation and substituting value we get:

 Average acceleration of the car during the 5.6 s is 5 m/s²
Average acceleration of the car during the 5.6 s is 5 m/s²
b. By using
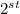 kinematic equation and substituting value we get:
kinematic equation and substituting value we get:
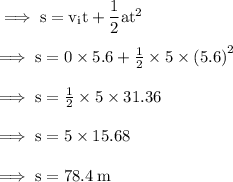
 Distance travelled by car in 5.6 s is 78.4 m
Distance travelled by car in 5.6 s is 78.4 m