the slope goes by several names
• average rate of change
• rate of change
• deltaY over deltaX
• Δy over Δx
• rise over run
• gradient
• constant of proportionality
however, is the same cat wearing different costumes.
let's do a), c) and last b).
a)

c)
well, we know it passes through (7 , 13) and we know its slope, so let's use that
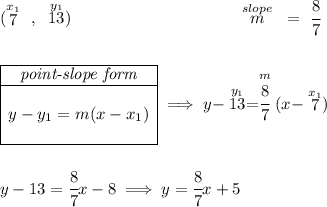
b)
another point? well, hmmm let's pick a random "x" value hmmm say 7/8, so
![y=\cfrac{8}{7}x+5\qquad \qquad \boxed{x=\cfrac{7}{8}}\hspace{3em}y=\cfrac{8}{7}\stackrel{x}{\left( \cfrac{7}{8} \right)}+5\implies y=1+5\implies \boxed{y=6} \\\\[-0.35em] ~\dotfill\\\\ ~\hfill \stackrel{another~point}{{\Large \begin{array}{llll} \left((7)/(8)~~,~~6 \right) \end{array}}}~\hfill](https://img.qammunity.org/2023/formulas/mathematics/high-school/wixftazg55rumpjli0fqpxxh2icfnx71kp.png)