Question

Help to find the answer!

Use the 45º-45º-90º triangle theorem to find the length of the hypotenuse of a right triangle if the legs are 15√2.

Answer 1

Let's consider the given triangle be ∆ABC. First of all we must know the unique property of triangle 45° - 45° - 90°. In this the ratio of sides of the triangle is given by,

`\implies` a : b : c = 1 : 1 : √2

Now as per your question elaborating it by placing values of sides in our ∆ABC,

Where,

• c = hypotenuse
• a & b = two sides

`:\implies\sf{c = 15 √(2) * √(2) }`

`:\implies\sf{c = 15 * √(2) * √(2) }`

`:\implies\sf{c = 15 * 2}`

`:\implies\sf{c = 30}`

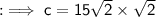
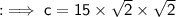
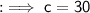
• The length of hypotenuse is 30cm.

Answer 2

• 30 units

Explanation:

The ratio of sides in 45º-45º-90º triangle is:

• a : b : c = 1 : 1 : √2

If both a and b are 15√2, the hypotenuse is:

• c = 15√2*√2 = 15*2 = 30