Question

Find the solution of the system of equations.
-6x-10y=-18 -3x+10y=36

Answer 1

problem one

x = 3- 5y/3

y = 9/5 - 3x/5

problem 2

x= -12 + 10y/3

y= 18/5 + 3x/10

Explanation:

Answer 2

The solution to the system of equation -6x-10y=-18 and -3x+10y=36 shows that the value of x = -2 and y = 3

What is a system of linear equation?

A system of linear equation is an algebraic equations that is written in the form Ax + By = C. It consist of two equation and two variable ( x and y) and a constant.

Using the substitution method to solve the system of equation:

-6x - 10y = -18 --- (1)

-3x + 10y = 36 ---- (2)

From (1);

-6x - 10y = - 18

-6x - 10y + 10y = -18 + 10y

-6x = 10y - 18

`x = (-5)/(3)y + 3`

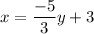
Replace the value of x into equation (2)

-3x + 10y = 36

`-3((-5)/(3)y+3) + 10y = 36`

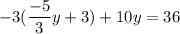
15y - 9 = 36

15y = 45

y = 45/15

y = 3

Substitute y = 3 into the equation
`x = (-5)/(3)y + 3`

`x = (-5)/(3)(3) + 3`

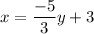
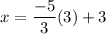
x = -2