Question

What is the slope of -x+ 5y = 10?

Answer 1

`\textsf{Slope}=(1)/(5)`

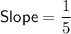
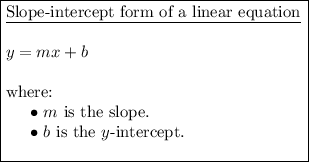
Explanation:

`\boxed{\begin{minipage}{6.3 cm}\underline{Slope-intercept form of a linear equation}\\\\$y=mx+b$\\\\where:\\ \phantom{ww}$\bullet$ $m$ is the slope. \\ \phantom{ww}$\bullet$ $b$ is the $y$-intercept.\\\end{minipage}}`

Given equation:

`-x+5y=10`

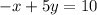
To find the slope of the given equation, use algebraic operations to isolate y.

Add x to both sides of the equation:

`\implies -x+5y+x=10+x`

`\implies 5y=x+10`

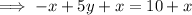
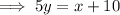
Divide both sides of the equation by 5:

`\implies (5y)/(5)=(x+10)/(5)`

`\implies y=(1)/(5)x+(10)/(5)`

`\implies y=(1)/(5)x+2`

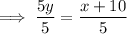
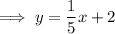
The coefficient of x is the slope of the equation.

Therefore, the slope of the given equation is ¹/₅.

Answer 2

Slope = 1/5

Explanation:

Given equation,

→ -x + 5y = 10

The slope-intercept form,

→ y = mx + b

→ slope = m

Now the slope-intercept form is,

→ -x + 5y = 10

→ 5y = x + 10

→ y = (x + 10)/5

→ [ y = (1/5)x + 2 ]

Then the required slope will be,

→ y = (1/5)x + 2

→ Slope = m = 1/5

Hence, the required slope is 1/5.