Answer:
1. 1 second.
2. No.
Explanation:
Given function:
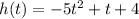
where:
- h is the height of the ball (in meters).
- t is the time (in seconds).
Question 1
The ball will hit the ground when the height is zero.
Substitute h = 0 into the given function and solve for t:
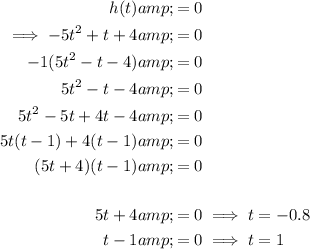
As t ≥ 0, t = 1 only.
Therefore, the ball will hit the ground after 1 second after it is thrown in the air.
Question 2
The x-coordinate of the vertex of a quadratic function is:
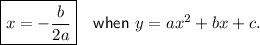
Therefore, the x-coordinate of the vertex of the given function is:

Substitute t = 0.1 into the function to find the maximum height of the ball:
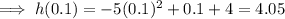
Therefore, the ball will not reach a height of 5 m, as the maximum height it can reach is 4.05 m, and 5 > 4.05.