Given the Rational Function:
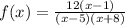
(1.1) You can rewrite it in this form:
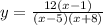
• By definition, the x-value is zero when the function intersects the y-axis. Therefore, you need to substitute this value into the equation and then evaluate, in order to find the y-intercept:

Then, you get:
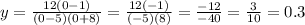
As an ordered pair, this is:
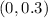
• In order to find the x-intercept, you need to substitute this value into the function and solve for "x", because the y-value is zero when the function intersects the x-axis:

Then, you get:
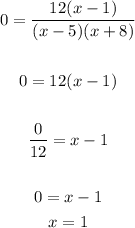
As an ordered pair, this is:
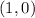
• In order to find the Vertical asymptote, you need to make the denominator equal to zero and solve for "x":
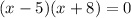
Notice that you get two Vertical Asymptotes:
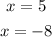
(1.2) By definition, the Domain of a function is the set of all the possible input values for which the function is defined.
Rational Functions are not defined for those numbers that make the denominator equal to zero. If you make the equation equal to zero and solve for "x", you get:
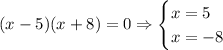
Therefore:
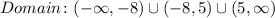
By definition, the Range is the set of all the possible output values of the function. For Rational Functions, the Range of the Rational Function is the Domain of its inverse.
In this case, you can find the inverse of the given function as follows.
- Solve for "x":
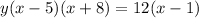

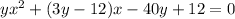
Applying the Quadratic Formula:
![x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2023/formulas/mathematics/college/rxvf73usjbbwyik14knxdemoz21vfz2ufc.png)
Where:
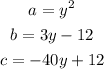
You get:
![x=\frac{-(3y-12)\pm\sqrt[]{(3y-12)^2-4(y^2)(-40y+12)}}{2\cdot y^2}](https://img.qammunity.org/2023/formulas/mathematics/college/aj2czd3lig0s5o38vkr28gqv21iuxolhll.png)
![x=\frac{-(3y-12)\pm\sqrt[]{(3y-12)^2-4(y^2)(-40y+12)}}{2y^2}](https://img.qammunity.org/2023/formulas/mathematics/college/10bp7nbhs2f8v2zxnm5aog74viabt8vq9c.png)
- Interchange the variables:
![y=\frac{-(3x-12)\pm\sqrt[]{(3x-12)^2-4(x^2)(-40x+12)}}{2x^2}](https://img.qammunity.org/2023/formulas/mathematics/college/8bz9tcpmmwnspv3n0qzk6y0hzoliluo4x9.png)
Notice that:
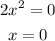
Hence, the Range of the original function is:
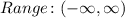
(1.3) By definition, if the degree of the numerator is less than the degree of the denominator, the Horizontal Asymptote is:

In this case, you can identify that the degree of the numerator is 1 and the degree of the denominator is 2:
Therefore, the Horizontal Asymptote is:

By definition, the Horizontal Asymptote indicates the end behavior of the Rational Function. In this case, it indicates that:
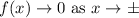
(1.4) Knowing all the data of the function, you can graph it:
Hence, the answers are:
(1.1) x-intercept:
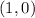
y-intercept:
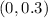
Vertical Asymptotes:
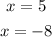
(1.2) Domain:
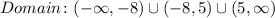
Range:
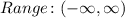
(1.3)
Other Asymptote:
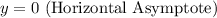
End Behavior:
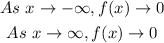
(1.4)