Question

Ises 12.4. plete the following: Graph the vertex, focus, and endpoints of the latus rectum; then draw the parabola for each equation in problem 1. Find the intercepts and domain and perform the symmetry test on each parabola with equation: (c) y'= -4.x (a) y'=&r (d) x - 4y

Answer 1

The given parabola is

`y^2=-4x`

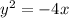
The vertex of this parabola is (0,0), the origin of the coordinate system.

The focus is determined by p. We know that 4p = -4, which means p = -1.

So, the focus is (-1, 0).

The latus rectum passes through the focus perpendicularly, which means its ending points are (-1, 2) and (-1, -2).

The parabola is shown in the image below.