Question

Solve for x assuming the expression is set to 0:

Answer 1

Step-by-step explanation

Given:

`-x^2-2x-3=0`

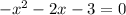
Then, we can multiply the whole expression times -1:

`x^2+2x+3=0`

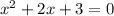
Next, we can find the solution for x using the General Quadratic Formula:

`x_(1,2)=(-b\pm√(b^2-4ac))/(2a)`

where a, b and c are the coefficients of the quadratic equation in the form ax² + bx + c = 0. Thus, considering that in our case a = 1, b = 2 and c = 3:

`x_(1,2)=(2\pm√(2^2-4(1)(3)))/(2(1))`
`x_(1,2)=(2\pm√(4-12))/(2)`
`x_(1,2)=(2\pm√(-8))/(2)`

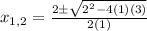
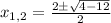
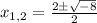
Finally, calculating the two solutions: