Question

Use the formula for continuous compounding to compute the balance in the account after 1, 5, and 20 years. Also, find the APY for the account. $4,000 deposit in an account with an APR of 8%.The balance in the account after 1 year is approximately $  (Round to the nearest cent as needed.)

Answer 1

Given:

`\begin{gathered} P=4000 \\ r=8\% \\ t=1year \end{gathered}`

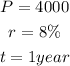
Required:

To find the balance amount after 1 year.

Step-by-step explanation:

Now consider the formula

`\begin{gathered} A=Pe^(rt) \\ \\ =4000(e^(0.08*1)) \\ \\ =4000(2.71828)^(0.08) \\ \\ =4333.15 \end{gathered}`

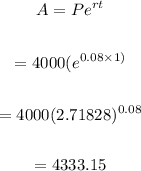
The total amount accrued, principal plus interest, with compound interest on a principal of $4,000.00 at a rate of 8% per year compounded continuously over 1 years is $4,333.15.

Final Answer:

The balance amount after 1 year is $4,333.2