First, lets remember that a paraboloid is just a parabola that was rotated around the Y axis. So lets find the equation from our parabola that is been rotated. We know that the focus is in the point (0,5), so we can find our equation:
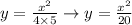
Just to remember, a parabola that has focus in (0,P) has equation as:
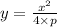
We can draw our parabola:
So we want to find the width knowing that the depth is 2 feet our 24 inches. That means that he wants to know the X that make the Y be 24, so lets find it:
![y=(x^2)/(20)\rightarrow x^2=24*20\rightarrow x=\pm\sqrt[]{480}\rightarrow x^(\prime)\cong21.908\text{ and }x\~\~^{}\cong-21.908](https://img.qammunity.org/2023/formulas/mathematics/college/8vlq4n9onw9p34a7gokl2j2r63gcgt7q70.png)