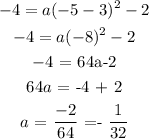Question

Below is the graph of a parabola with its vertex and another point on the parabola labeled.Write an equation of the parabola.• (-2,3)• (-4,-5)

Answer 1

The equation of the parabola is;

`x\text{ = -}(1)/(32)(y-3)^2-2`

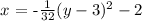
Here, we want to write the equation of the parabola

As we can see, for this kind of parabola, the x coordinate becomes the independent variable while the y coordinate becomes the dependent variable

We have the vertex as (-2,3) and the other point as (-4,-5)

We have the general equation form as;

`x\text{ = }a(y-k)^2\text{ + }h`

where (h,k) is the vertex of the parabola

From the given question, h = -2 and k is 3

Susbtituting these values;

`x=a(y-3)^2-2`

To get the value of a, we substitute the coordiantes of the other point;

We have this as;

`\begin{gathered} -4=a(-5-3)^2-2 \\ -4=a(-8)^2-2 \\ -4\text{ = 64a-2} \\ 64a\text{ = -4 + 2} \\ a\text{ = }(-2)/(64)\text{ =- }(1)/(32) \end{gathered}`