The percentage of values between 25 and 34 is 81.5%
Here, we want to get the percentage of data values in the given interval
To do this, we proceed as follows;
Firstly, we will need to get the z-values for each of the points
To do this, we are going to use the z-score formula;

Now, we have to find the probability between the two z-scores
We have this as the percentage of values from two standard deviation below the mean to 1 standard deviation above the mean
We can get this from the normal distribution curve;
The addition of the values here are;
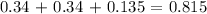
Writing this probability as a percentage, we have 81.5%