Consider that the Volume of a cone (V), which has a height (h) and radius (r) is given by,
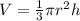
Figure-1
Given that the radius is 8 in. and the height is 14 in. the volume is calculated as,
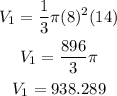
So the volume of this cone is 938.289 cubic inches approximately.
Figure-2
Given that the radius is 9 in. and the height is 13 in. the volume is calculated as,
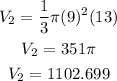
So the volume of this cone is 1102.699 cubic inches approximately.
Figure-3
Given that the radius is 7 in. and the height is 10 in. the volume is calculated as,
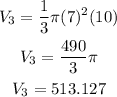
So the volume of this cone is 513.127 cubic inches approximately.
Figure-4
Given that the radius is 6 in. and the height is 12 in. the volume is calculated as,
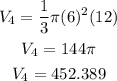
So the volume of this cone is 1102.699 cubic inches approximately.
Observe the volume values obtained for all four cones,
[tex]\begin{gathered} 452.389<513.127<938.289<1102.699 \\ \Rightarrow V_4Thus,
the order of least to greatest volume is, figures 4, 3, 1, 2 respectively.