90720 distinguishable permutations.
1) Considering that the word CLEVELAND, actually has 9 letters and there is the repetition of E, L.
2) And the question makes no comment about restraining repetitions we can write considering the repetition formula:
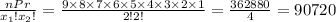
In this formula above, we can place the repeated letters (2 letters E and L) as x_1 and x_2, and on the numerator the number of letters, in this case, 9.
3) So there are 90720 possibilities of distinguishable permutations