The point P divide the line segement AB in 2:3 ratio.
The point are A(-3,-10) and B(2,0).
The formula for the coordinates of the point that divide the segment A(x_1,y_1) and (x_2,y_2) in ratio m:n.
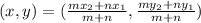
For the given problem m = 2 and n = 3.
Substitute the given values in the formula to obtain the coordinates of point P.

So coordinates of point P is (-1,-6).