Solution:
Let x represent one of the investments
Since the total of the two investments is $56,000, the other investment will be
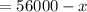
If x is invested at 4% and the other is invested at 2%, then, it can be expressed as
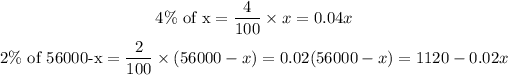
And, their income is $1400, then, it can be expressed as

Solve for x

The other investment will be
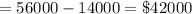
Hence,
At a 4% rate, the investment is $14000 and at a 2% rate, the investment is $42000