Question

A stuntman drives a car with a mass of 1600 kg on a drawbridge. The car accelerates with a constant force of 8000 N. While he is driving, the drawbridge is raised to an incline of 30°. What is the car's new acceleration on this incline, ignoring the force due to air resistance? (recall that g=9.8 m/s^2)

Answer 1

Given:

• Mass of car, m = 1600 kg

,

• Force, N = 8000 N

,

• Angle, θ = 30 degrees

Let's find the car's new acceleration on this angle ignoring the force due to air resistance.

First apply the formula which shows the weight of the car that is parallel to this parallel to this angle:

`w=mgsin\theta`

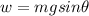
Where:

m - 1600 kg

g is acceleration due to gravity = 9.8 m/s²

θ = 30 degrees

Plug in values and solve for w:

`\begin{gathered} w=mgsin\theta \\ \\ w=1600*9.8*sin30 \\ \\ w=7840\text{ N} \end{gathered}`

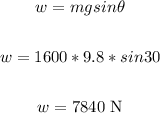
Now, the weight of the car parallel to the angle is 7840 N.

To find the acceleration, apply Newton's Second Law of motion:

ΣF = ma

F = ma + w

Where a is the acceleration.

Rewrite the formula for a and solve:

`a=(F-w)/(m)`

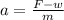
Plug in values and solve for a:

`\begin{gathered} a=(8000-7840)/(1600) \\ \\ a=(160)/(1600) \\ \\ a=0.1\text{ m/s}^2 \end{gathered}`

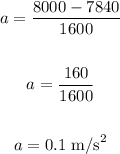
Therefore, the new acceleration of the car is 0.1 m/s².

ANSWER:

0.1