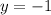Find the equation of a line with given slope and containing the given point. Write the equation in slope-intercept form. Horizontal line containing (4,-1)Y=

Question

asked Jul 7, 2023 41.8k views

1 Answer

Neberaa · Answer 1 · 2023-07-11T10:25:33+0000

Solution

Since,Horizontal line containing (4,-1) then the slope (m) is zero (0)

The equation of the line in Slope-Intercept form is:

Where "m" is the slope and "b" is the y-intercept.

We know that this is a horizontal line.

This means that: m=0

Knowing that this line contains the point (4,-1) we can substitute this coordinates m=0 into y = mx + b, and then we solve for b

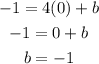
$\begin{gathered} -1=4(0)+b \\ -1=0+b_{} \\ b=-1 \end{gathered}$

Therefore, b = -1

Therefore, the equation of this line in Slope-intercept form is:

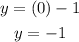
$\begin{gathered} y=(0)-1 \\ y=-1 \end{gathered}$

The final answer