Answer:
-1
Step-by-step explanation:
Given the points 6, -12) and (15, -3).
The slope of the line joining the two points is calculated below:
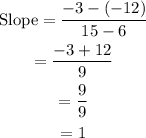
Next, we determine the perpendicular slope.
Definition: Two lines are perpendicular if the product of their slopes is -1.
Let the perpendicular slope = m
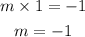
The perpendicular slope is -1.