Question

Jane has been saving quarters and dimes. She opened up the piggy bank and determined that it contained 35 coins worth $6.80. Determine how many dimes and quarters were in the piggy bank.

Answer 1

Hello!

First of all, let's write some important information:

• There are 35 coins in total;

,

• The total amount is $6.80.

Let's write d for dimes and q for quarters.

Writing the exercise as a linear system, we will have:

`\begin{cases}d+q={35} \\ 0.10d+0.25q={6.80}\end{cases}`

Let's isolate the variable d in the first equation:

`\begin{cases}d+q={35\rightarrow d=35-q} \\ 0.10d+0.25q={6.80}\end{cases}`

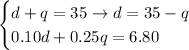
Now let's replace it in the second equation:

`\begin{gathered} 0.10d+0.25q=6.80 \\ 0.10(35-q)+0.25q=6.80 \\ 3.50-0.10q+0.25q=6.80 \\ 0.15q=6.80-3.50 \\ 0.15q=3.30 \\ q=(3.30)/(0.15) \\ \\ \boxed{q=22\text{ quarter coins}} \end{gathered}`

As we know the number of quarters, we just have to replace it:

`\begin{gathered} d+q=35 \\ d+22=35 \\ d=35-22 \\ \boxed{d=13\text{ dimes coins}} \end{gathered}`

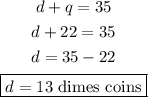
Answer:

In the pig bank were 13 dimes coins and 22 quarter coins.