Question

The width of a rectangle is 3 less than twice the length, x. If the perimeter of the rectangle is 36 feet. Find length and width.

Answer 1

The length of the rectangle is given to be x.

The width of a rectangle is 3 less than twice the length. This can be written mathematically to be:

`\begin{gathered} \text{Twice the length }\Rightarrow2x \\ \text{Three less }\Rightarrow2x-3 \end{gathered}`

Therefore, we will have the rectangle to look as shown below:

The formula to calculate the perimeter of a rectangle is given to be:

`P=2(l+w)`

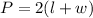
Given that we have the following parameters:

`\begin{gathered} P=36 \\ l=x \\ w=2x-3 \end{gathered}`

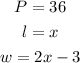
Substituting these values, we can get the value of x as shown below:

`\begin{gathered} 36=2(x+2x-3) \\ 36=2(3x-3) \\ 36=6x-6 \\ 6x=36+6 \\ 6x=42 \\ x=(42)/(6) \\ x=7 \end{gathered}`

Given that the length has been calculated, we can get the width to be:

`\begin{gathered} w=2(7)-3 \\ w=14-3 \\ w=11 \end{gathered}`

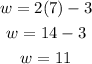
ANSWER

The length of the rectangle is 7 feet and the width of the rectangle is 11 feet.