SOLUTION
From
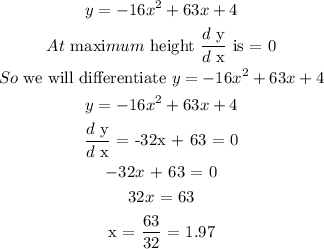
Now, we will substitute 1.97 for x into y

Since y which is the height of the arrow is 66.02 feet, and the tree is 68 feet tall, the arrow can not pass over the tree. Because for the arrow to pass over the tree, its height must be above 68 feet tall.