Question

4. The length of a rectangle is 3 more than the width. If the area is 40 square inches, what are the dimensions? O width = 8, length = 5 O width = 5, length = 8 0 width = -8, length = 5 O width = -5, length = 8

Answer 1

Answer:

Since the area is 40

width = 5, length = 8

Step-by-step explanation:

Let the length of the triangle be l, and the width be w.

Given that the length is 3 more than the width, we have:

l = w + 3 ...........................................................(1)

The area is given to be 40 square inches.

The formula for the area is:

A = lw

Therefore,

lw = 40 ................................................................(2)

Substitute the expression for l in (1) into (2)

w(w + 3) = 40

`w^2+3w-40=0`

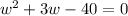
Solving the above, we have:

`\begin{gathered} (w+8)(w+5)=0 \\ w=8 \\ OR \\ w=5 \end{gathered}`

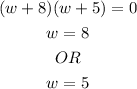
If w = 8, then

l = 8 + 3 = 11

If w = 5, then

l = 5 + 3 = 8

Since the area is 40, w = 5, l = 8 are the appropriate answers.