Given:
The volume of the can, V=36.
The diameter of the can, D=4.
The can has the shape of a cylinder.
The radius of the can is,
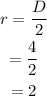
The equation for the volume of a cylinder is,

Here, h is the height of the cylinder.
The volume of a cone that fits perfectly inside a cylinder has the same radius and the same height as the cylinder
The equation for the volume of a cone is,
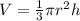
Therefore, the volume of the cone is 1/3 rd of the volume of the cylinder.
Hence, the volume of the cone can be found as,
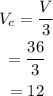
Therefore, the volume of the cone is 12.