Question

A car has a mass of 1,703 kg and an engine capable of producing 18,905 watts of power. If the engine runs at maximum power how fast will it take the car to go from 0 m/s to 10 m/s? (Round your answer to the nearest tenth of a second).

Answer 1

Given

The mass is

`m=1,703\text{ kg}`

The power is

`P=18,905\text{ W}`

The initial velovcity is

`u=0\text{ m/s}`

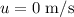
The final velocity is

`v=10\text{ m/s}`

To find

Time taken by the car to go from 0 m/s to 10 m/s.

Step-by-step explanation

By work-energy theorem we have that

Work done is equal to the change in kinetic energy.

Thus,

`W=(1)/(2)m\left(v^2-u^2\right?`

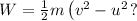
Again work done can be written as

`W=P* t`

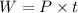
where t is the time

Equating the two equations we have,

`\begin{gathered} P* t=(1)/(2)m\left(v^2-u^2\right? \\ \Rightarrow18,905t=(1)/(2)*1703*10^2 \\ \Rightarrow t=4.504\text{ s} \end{gathered}`

Conclusion

The time taken is 4.504 s