Question

200 lottery tickets are sold for $6 each. The person with the single winning ticket will get $90. What is the expected value for a ticket in this lottery?

Answer 1

The probability of winning is 1/200.

The probability of losing is 199/200.

The gain of winning is

($90 - $6) that is $90 of the winning ticket minus the $6 ticket cost.

The loss of lossing is -$6 that is a player loses $6 for the ticket.

The expected value therefore is calculated as

`\begin{gathered} E(X)=(90-6)((1)/(200))+(-6)((199)/(200)) \\ E(X)=(84)((1)/(200))+(-6)((199)/(200)) \\ E(X)=0.42-5.97 \\ E(X)=-5.55 \end{gathered}`

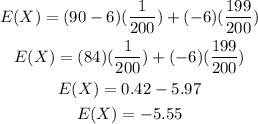
Therefore, the expected value for a ticket in this lotter is -$5.55 or an average loss of $5.55.