Given:
The initial velocity of the object is: u = 13 cm/s
The time taken by an object to change the position coordinate is: t = 2.95 s
The change in the position (displacenent) is: Δx = -5 cm - 3.18 cm = - 1.82 cm
To find:
The acceleration of an object.
Step-by-step explanation:
The acceleration of an object can be determined by using following kinematical equation,
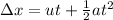
Rearranging the above equation, we get:
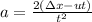
Substituting the values in the above equation, we get:

Final answer:
The acceleration of an object is -8.9351 cm/s^2.