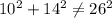Question

Triangle ABC has side lengths 10 14 and 26 do the silence form a Pythagorean triple explain

Answer 1

Given the side lengths of a triangle ABC;

`a=10,b=14,c=26`

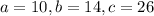
The side lengths form a Pythagorean triple if ithe square of the longest side is equal to the sum of squares of the remaining two sides.

Thus;

`\begin{gathered} 10^2+14^2=100+196 \\ \\ 10^2+14^2=296 \\ \\ 296\\e29^2 \\ \\ \text{ Thus;} \\ \\ 10^2+14^2\\e26^2 \end{gathered}`

Hence, they do not form a Pythagorean triple.

CORRECT OPTION: (B) No, they do not form a Pythagorean triple.

`10^(2)+14^(2)\\e26^(2)`