Answer:
Step-by-step explanation:
A.
The standard form of a quadratic equation is given as;

Given the below quadratic equation;
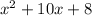
If we compare the given quadratic equation with the standard form of a quadratic equation, we can see that;
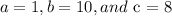
B.
We can tell if the graph of the given equation will open up or down by considering the coefficients of x^2.
If the coefficient of x^2 is greater than zero, then the parabola will open upwards but if the coefficient of x^2 is less than zero, then the parabola will open downwards.
Since the coefficient of x^2 in the given equation is greater than zero, then the parabola will open upwards.
C.
To find t