Question

Find the value of x and list the sides of ABC in order from shortest to longest of the indicated measures. (Hint: Find the angle measures first, then decide which sic longest.)

Answer 1

To solve this problem, we have to remember the triangle sum theorem, that says that the sum of the interior angles of a triangle is 180°. To find x, sum the expressions for each angle and make it equal to 180, this way

`\begin{gathered} \measuredangle A+\measuredangle B+\measuredangle C=180 \\ 9x+29+93-5x+10x+2=180 \\ 14x+124=180 \\ 14x=180-124 \\ 14x=56 \\ x=(56)/(14) \\ x=4 \end{gathered}`

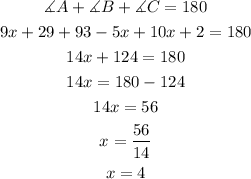
With this value, find the measure of each angle.

`\begin{gathered} \measuredangle A=9x+29=9\cdot4+29=65 \\ \measuredangle B=93-5x=93-5\cdot4=73 \\ \measuredangle C=10x+2=10\cdot4+2=42 \end{gathered}`

Finally, let's remember this: the wider the angle, the longer is its opposite side. It means, the ordered sides from shortest to longest are:

AB (opposite to angle C)

BC (opposite to angle A)

AC (opposite to angle B)