Question

Mass of Car (kg)0.036Displacement in (m)1.05Trial Time (t) in (s)1 0.732 0.723 0.73Average Time0.73a. calculate gravitational potential energyb. calculate average speedc. calculate final speed d. calculate kinetic energye. calculate efficiency

Answer 1

We will have the following:

a. We will determine the gravitational potential energy as follows:

`\begin{gathered} U_g=(0.036kg)(9.8m/s^2)(1.05m)\Rightarrow U_g=(1323)/(2500)J \\ \\ \Rightarrow U_g=0.5292J \end{gathered}`

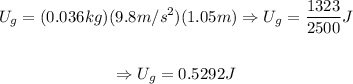
So, the gravitational potential energy is 0.5292 Joules.

b. Average speed:

`\begin{gathered} v_a=(1.05m)/(0.73s)\Rightarrow v_a=(105)/(73)m/s \\ \\ \Rightarrow v_a\approx1.44m/s \end{gathered}`

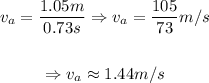
So, the average speed was 105/73 m/s, that is approximately 1.44 m/s.

c. The final speed will be determined as follows:

`v_f=(9.8m/s^2)(0.73s)\Rightarrow v_f=7.154m/s`

So, the final velocity will be 7.154 m/s.

d. The kinetic energy will be:

`\begin{gathered} k=(1)/(2)(0.036kg)(7.154m/s)^2\Rightarrow k=0.921234888...J \\ \\ \Rightarrow k\approx0.92J \end{gathered}`

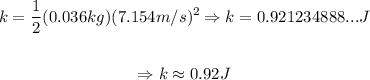
So, the kinetic energy is approximately 0.92 Joules.

e. We will determine the efficiency as follows:

`\begin{gathered} E=(0.92J)/(0.5292)\ast100\Rightarrow E=173.8373167... \\ \\ \Rightarrow E\approx173.84 \end{gathered}`

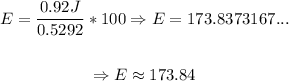
So, the efficiency is approximately 173.84%.