Question

Find the width and area based on the length being 11 and the perimeter being 34

Answer 1

the width is 6 units

Area: 66 square units

Step-by-step explanation

Step 1

the perimeter of a rectangle is given by.

`P=2(l+w)`

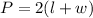
where w is the width and l is the length

then

Let

length= 11

width= unknonwn= w

perimeter= 34

replace in the formula and solve for w

`\begin{gathered} P=2(l+w) \\ \text{break the parenthesis} \\ P=2l+2w \\ \text{replace} \\ 34=2(11)+2w \\ 34=22+2w \\ \text{subtract 22 in both sides} \\ 34-22=22+2w-22 \\ 12=2w \\ \text{divide both sides by 2} \\ (12)/(2)=(2w)/(2) \\ 6=w \end{gathered}`

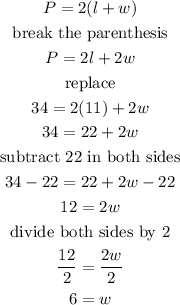
Step 3

Area

the area of a rectangle is given by

`\begin{gathered} \text{Area}=\text{ length}\cdot width\text{ } \\ \text{replace} \\ \text{Area}=11\cdot6 \\ A=66(un)^2 \end{gathered}`

therefore, the width is 6

Area: 66 square units

I hope this helps you