In the question, the length of the rectangle is 11 mm and the breadth is given as 5cm. This also serves as the base and the height of the triangle respectively.
Step-by-step explanation
The steps to take are;
1) Find the area of the rectangle and the triangle
2) Find the area of the two ratios.
But we must make the units of the length and the breadth of the rectangle equal. Therefore
![\begin{gathered} \text{Since 10mm = 1cm} \\ 11\operatorname{mm}=\frac{\text{11}}{10}cm=1.1\operatorname{cm} \end{gathered}]()
Hence the areas are as follows:
![\begin{gathered} \text{Area of a triangle = }(1)/(2)*\text{base x height = 0.5 x 1.1 x5} \\ =2.75\operatorname{cm} \\ \text{Area of a rectangle = Length x breadth = 1.1 x 5}=\text{ 5.5}cm^2 \\ \end{gathered}]()
Therefore the ratio of the area of the triangle to the area of the rectangle is given as
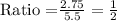
Answer: Option C