Question

How would you write a system of equations to represent the line and estimate the solution?

Answer 1

a)

We have the graph of two lines and we must write a system of equations to represents the two lines.

To write the system of equations we must find the equation for each line:

We need to use that the equation for a line is y = mx + b, where m is the slope and b is the y-intercept.

L1:

We can see that the line intercepts the y-axis when y = 3. So we have

`y=mx+3`

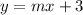
Now, we need to replace in the equation on the points which the line passes and then solve it for m. in this case we can take the point (2, 2)

Replacing in the equation,

`\begin{gathered} 2=m(2)+3 \\ 2-3=m(2) \\ (-1)/(2)=m \\ m=-(1)/(2) \end{gathered}`

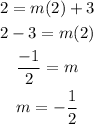
Then, the equation for L1 would be

`\begin{gathered} y=-(1)/(2)x+3 \\ \text{ Rewritin,} \\ (1)/(2)x+y=3 \end{gathered}`

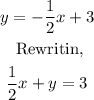
L2:

We can see that the line intercepts the y-axis when y = -1. So we have

`y=mx-1`

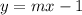
Now, we need to replace in the equation on the points which the line passes and then solve it for m. in this case we can take the point (1, 1)

Replacing in the equation,

`\begin{gathered} 1=m(1)-1 \\ 1+1=m(1) \\ (2)/(1)=m \\ m=2 \end{gathered}`

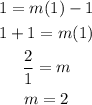
Then, the equation for L2 would be

`\begin{gathered} y=2x-1 \\ \text{ Rewriting,} \\ -2x+y=-1 \end{gathered}`

Knowing the two equations we can write a system of equations:

`\begin{cases}(1)/(2)x+y=3 \\ -2x+y=-1\end{cases}`

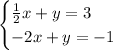
b)

We need to estimate the solution of the system using the graph.

The solution of the system is the point where the two lines intersect them, so seeing the graph we can estimate that this point is (1.6 , 2.2)

ANSWER:

a)

`\begin{cases}(1)/(2)x+y=3 \\ -2x+y=-1\end{cases}`

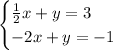
b) (1.6 , 2.2)