EXPLANATION
The probability that the fraction will be less than or equal to 3/2 is given by the following relationship:
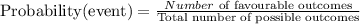
Now, we can list all the possible fractions that we could get:
Here are the combinations with 4 as the numerator:
4 / 4
4 / 6
4 / 5
4 / 3
Here are the combinations with 6 as the numerator:
6/ 4
6 / 6
6 / 5
6 / 3
Here are the combinations with 5 as the numerator:
5/ 4
5 / 6
5 / 5
5 / 3
Here are the combinations with 3 as the numerator:
3/ 4
3 / 6
3 / 5
3 / 3
As we can see, the are 4*4= 16 different combinations that we could get.
Now, we need to define wich possible combinations are less or equal than 3/2=1.5.
4 / 4 = 1 [LESS THAN 3/2]
4 / 6 = 2/3 = 0.67 [LESS THAN 3/2]
4 / 5 = 0.8 [LESS THAN 3/2]
4 / 3 = [LESS THAN 3/2]
-------------
6/ 4 = 3/2 [EQUAL THAN 3/2]
6 / 6 = 1 [LESS THAN 3/2]
6 / 5 = [LESS THAN 3/2]
6 / 3 [NO --> GREATER THAN 3/2]
------------
5/ 4 = 1.25 [LESS THAN 3/2]
5 / 6 [LESS THAN 3/2]
5 / 5 [LESS THAN 3/2]
5 / 3 [NO -->GREATER THAN 3/2]
------------
3/ 4 [LESS THAN 3/2]
3 / 6 [LESS THAN 3/2]
3 / 5 [LESS THAN 3/2]
3 / 3 [LESS THAN 3/2]
As we can see, there are 14 possible combinations that are less or equal to 3/2.
Hence,
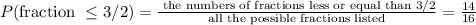
Simplifying:
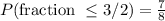
The probability will be 7/8