A linear equation in the slope-intercept form is y = mx + b, where m is the slope and b is the y-intercept.
Given two points (x₁, y₁) and (x₂, y₂), the slope (m) can be calculated:

So, to find the slope and the equation of the line, follow the steps below.
Step 01: Choose two points from the table and calculate "m".
Let's choose the points (0, 8) and (2, 14).
Substituting them in the equation:
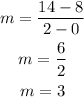
The slope is 3.
Step 02: Choose one point and substitute in the equation of the line to find b.
Knowing that m = 3, the equation of the line is:
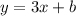
Choosing the point (0, 8) and substituting it in the equation:
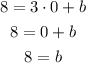
So, the equation is:
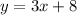
Answer:
The slope is 3.
The equation of the line is y = 3x + 8.