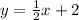Question

How do I write an equation of a line passing through

Answer 1

Answer:

The equation is:

`y=(1)/(2)x+2`

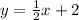
Step-by-step explanation:

Given the point (2, 3), and the equation:

y - 4 = -2(x + 3) .............................................(1)

`\begin{gathered} y=-2x-6+4 \\ =-2x-2 \end{gathered}`

Comparing with the equation of a line:

`y_{}=mx+b`

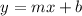
where m is the slope, and b is the y-intercept

The slope in (1) is -2

Note that perpendicular lines have their slopes as negative reciprocals of each other.

The negative reciprocal of -2 is 1/2

The equation of a line perpendicular to (1) is:

`y=(1)/(2)x+b`

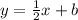
Applying the given point (2, 3), we have x = 2, y = 3

`\begin{gathered} 3=(1)/(2)(2)+b \\ \\ b=3-1=2 \end{gathered}`

The equation is therefore;

`y=(1)/(2)x+2`